Local Minima or Minimize

$bar{x}in :S$ is said to be local minima of a function $f$ if $fleft ( bar{x} right )leq fleft ( x right ),forall x in N_varepsilon left ( bar{x} right )$ where $N_varepsilon left ( bar{x} right )$ means neighbourhood of $bar{x}$, i.e., $N_varepsilon left ( bar{x} right )$ means $left | x-bar{x} right |

Local Maxima or Maximizer

$bar{x}in :S$ is said to be local maxima of a function $f$ if $fleft ( bar{x} right )geq fleft ( x right ), forall x in N_varepsilon left ( bar{x} right )$ where $N_varepsilon left ( bar{x} right )$ means neighbourhood of $bar{x}$, i.e., $N_varepsilon left ( bar{x} right )$ means $left | x-bar{x} right |

Global minima

$bar{x}in :S$ is said to be global minima of a function $f$ if $fleft ( bar{x} right )leq fleft ( x right ), forall x in S$

Global maxima

$bar{x}in :S$ is said to be global maxima of a function $f$ if $fleft ( bar{x} right )geq fleft ( x right ), forall x in S$

Examples

Step 1 − find the local minima and maxima of $fleft ( bar{x} right )=left | x^2-4 right |$

Solution −

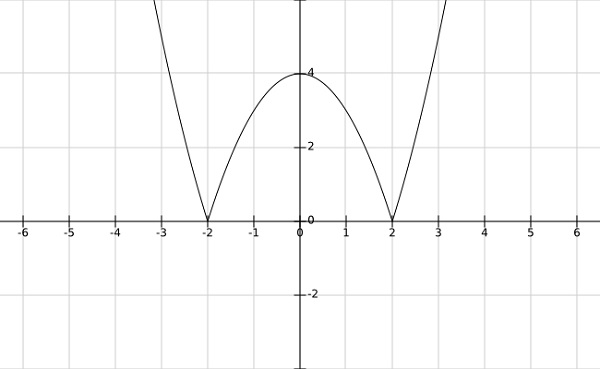
From the graph of the above function, it is clear that the local minima occurs at $x= pm 2$ and local maxima at $x = 0$

Step 2 − find the global minima af the function $fleft (x right )=left | 4x^3-3x^2+7 right |$

Solution −

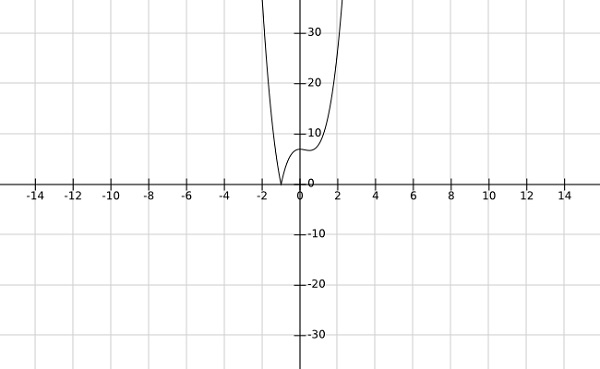
From the graph of the above function, it is clear that the global minima occurs at $x=-1$.