For electrical transformer, the EMF equation is a mathematical expression used to find the magnitude of induced EMF in the windings of the transformer.

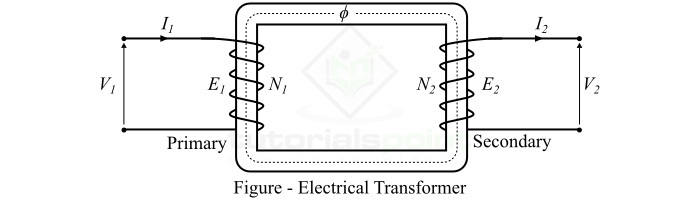
Consider a transformer as shown in the figure. If N₁ and N₂ are the number of turns in primary and secondary windings. When we apply an alternating voltage V₁ of frequency f to the primary winding, an alternating magnetic flux $phi$ is produced by the primary winding in the core.

If we assume sinusoidal AC voltage, then the magnetic flux can be given by,

$$mathrm{mathit{phi }:=:phi _{m}:mathrm{sin}:mathit{omega t}:cdot cdot cdot (1)}$$

Now, according to principle of electromagnetic induction, the instantaneous value of EMF e₁ induced in the primary winding is given by,

$$mathrm{mathit{e_{mathrm{1}}}:=:mathit{-N_{mathrm{1}}}frac{mathit{dphi }}{mathit{dt}}}$$

$$mathrm{Rightarrow mathit{e_{mathrm{1}}}:=:mathit{-N_{mathrm{1}}}frac{mathit{d}}{mathit{dt}}left ( phi _{m}: mathrm{sin}:mathit{omega t}right )}$$

$$mathrm{Rightarrow mathit{e_{mathrm{1}}}:=:mathit{-N_{mathrm{1}}}:mathit{omega phi :cos:omega t}}$$

$$mathrm{Rightarrow mathit{e_{mathrm{1}}}:=:-mathrm{2}mathit{pi fN_{mathrm{1}}}:mathit{phi_{m} :cos:omega t}}$$

Where,

$$mathrm{mathit{omega :=:mathrm{2}pi f}}$$

$$mathrm{because -mathit{cos:omega t}:=:mathrm{sin}left ( mathit{omega t-mathrm{90^{circ}}} right )}$$

Therefore,

$$mathrm{mathit{e_{mathrm{1}}}:=:mathrm{2}mathit{phi fN_{mathrm{1}}}:mathit{phi_{m}:mathrm{sin}left ( mathit{omega t-mathrm{90^{circ}}} right )}}:cdot cdot cdot (2)$$

Equation (2) may be written as,

$$mathrm{mathit{e_{mathrm{1}}}:=:mathit{E_{m_{mathrm{1}}}}mathrm{sin}left ( mathit{omega t-mathrm{90^{circ}}} right ):cdot cdot cdot (3)}$$

Where,$mathit{E_{m_{mathrm{1}}}}$ is the maximum value of induced EMF $mathit{e_{mathrm{1}}}$.

$$mathrm{mathit{E_{mathrm{m1}}}:=:mathrm{2}mathit{pi fN_{mathrm{1}}}:mathit{phi_{m}}}$$

Now, for sinusoidal supply, the RMS value $mathit{E_{mathrm{1}}}$ of the primary winding EMF is given by,

$$mathrm{mathit{E_{mathrm{1}}}:=:frac{mathit{E_{mmathrm{1}}}}{sqrt{2}}:=:frac{2mathit{pi fN_{mathrm{1}}}phi_{m}}{sqrt{2}}}$$

$$mathrm{thereforemathit{E_{mathrm{1}}}:=:4.44:mathit{fphi _{m}N_{mathrm{1}}}:cdot cdot cdot (4)}$$

Similarly, the RMS value E₂ of the secondary winding EMF is,

$$mathrm{mathit{E_{mathrm{2}}}:=:4.44:mathit{fphi _{m}N_{mathrm{2}}}:cdot cdot cdot (5)}$$

In general,

$$mathrm{mathit{E}:=:4.44:mathit{fphi _{m}N}:cdot cdot cdot (6)}$$

Equation (6) is known as EMF equation of a transformer.

For a given transformer, if we divide the EMF equation by the supply frequency, we get,

$$mathrm{frac{mathit{E}}{mathit{f}}:=:4.44:phi _{m}mathit{N}:=:mathrm{Constant}}$$

Which means the induced EMF per unit frequency is constant but it is not same on both primary and secondary side of the given transformer.

Also, from equations (4) and (5), we have,

$$mathrm{frac{mathit{E_{mathrm{1}}}}{mathit{E_{mathrm{2}}}}:=:frac{mathit{N_{mathrm{1}}}}{mathit{N_{mathrm{2}}}}:or:frac{mathit{E_{mathrm{1}}}}{mathit{N_{mathrm{1}}}}:=:frac{mathit{E_{mathrm{2}}}}{mathit{N_{mathrm{2}}}}}$$

Hence, in a transformer, the induced EMF per turn in the primary winding is equal to the induced EMF per turn in the secondary winding.

Numerical Example

A single phase 3300/240 V, 50 Hz transformer has a maximum magnetic flux of 0.0315 Wb in the core. Calculate the number of turns in primary and secondary windings.

Solution

Given data,

$$mathrm{mathit{E_{mathrm{1}}:=:mathrm{3300}:mathrm{V}:mathrm{and}:mathit{E_{mathrm{2}}:=:mathrm{240}:V}}}$$

$$mathrm{mathit{f}:=:50:Hz;:phi _{m}:=:0.0315:Wb}$$

The EMF equation of the transformer is,

$$mathrm{mathit{E}:=:4.44:mathit{fphi _{m}N}}$$

Therefore, for primary winding,

$$mathrm{mathit{N_{mathrm{1}}}:=:frac{mathit{E_{mathrm{1}}}}{4.44:mathit{fphi _{m}}}:=:frac{3300}{4.44times 50times 0.0315}}$$

$$mathrm{mathit{N_{mathrm{1}}}:=:471.9:=:472}$$

Also, for secondary winding,

$$mathrm{mathit{N_{mathrm{2}}}:=:frac{mathit{E_{mathrm{2}}}}{4.44:mathit{fphi _{m}}}:=:frac{240}{4.44times 50times 0.0315}}$$

$$mathrm{mathit{N_{mathrm{2}}}:=:34.32:=:35}$$

It is not possible for a winding to have part of a turn. Thus, the number of turns should be a whole number.