According to principle of electromagnetic induction, when the magnetic flux linking to a conductor or coil changes, an EMF is induced in the conductor or coil. In practice, the following two ways are used to bring the change in the magnetic flux linkage.

Method 1 − Conductor is moving in a stationary magnetic field

We can move a conductor or coil in a stationary magnetic field in such a way that the magnetic flux linking to the conductor or coil changes in magnitude. Consequently, an EMF is induced in the conductor. This induced EMF is known as dynamically induced EMF. It is so called because the EMF induced in a conductor which is in motion. Example of dynamically induced EMF is the EMF generated in the AC and DC generators.

Method 2 − A stationary conductor is placed in a changing magnetic field

When a stationary conductor or coil is placed in a moving or changing magnetic field, an EMF is induced in the conductor or coil. The EMF induced in this way is known as statically induced EMF. It is so called because the EMF is induced in a conductor which is stationary. The EMF induced in a transformer is an example of statically induced EMF.

Therefore, from the discussion, it is clear that the induced EMF can be classified into two major types namely,

• Dynamically Induced EMF

• Statically Induced EMF

Dynamically Induced EMF

As discussed in the above section that the dynamically induced EMF is one which induced in a moving conductor or coil placed in a stationary magnetic field. The expression for the dynamically induced EMF can be derived as follows −

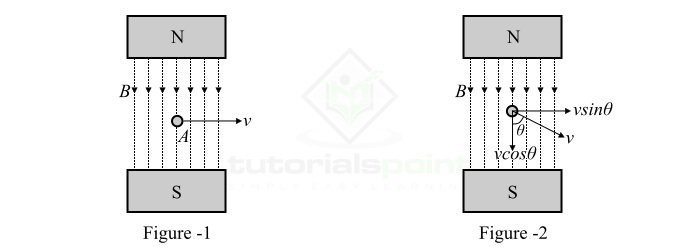
Consider a single conductor of length l meters located in a uniform magnetic field of magnetic flux density B Wb/m² as shown in Figure-1. This conductor is moving at right angles relative to the magnetic field with a velocity of v m/s.

Now, if the conductor moves through a small distance dx in time dt seconds, then the area swept by the conductor is given by,

$$mathrm{mathit{A:=:ltimes dx:}mathrm{m^{mathrm{2}}}}$$

Therefore, the magnetic flux cut by the conductor is given by,

$$mathrm{mathit{dphi }:=:mathrm{Flux:densitytimes Area: swept}}$$

$$mathrm{Rightarrow mathit{dphi }:=:mathit{Btimes ltimes dx}:mathrm{Wb}}$$

According to Faraday’s law of electromagnetic induction, the EMF induced in the conductor is given by,

$$mathrm{mathit{e}:=:mathit{N}frac{mathit{dphi }}{mathit{dt}}:=:mathit{N}frac{mathit{Bldx}}{mathit{dt}}}$$

Since, we have taken only a single conductor, thus N = 1.

$$mathrm{mathit{e}:=:mathit{Blv}:mathrm{volts}cdot cdot cdot (1)}$$

Where, v = dx/dt, velocity of the conductor in the magnetic field.

If there is angular motion of the conductor in the magnetic field and the conductor moves at an angle θ relative to the magnetic field as shown in Figure-2. Then, the velocity at which the conductor moves across the magnetic field is equal to “vsinθ”. Thus, the induced EMF is given by,

$$mathrm{mathit{e}:=:mathit{B:l:v}:mathrm{sinmathit{theta }}:mathrm{volts}cdot cdot cdot (2)}$$

Statically Induced EMF

When a stationary conductor is placed in a changing magnetic field, the induced EMF in the conductor is known as statically induced EMF. The statically induced EMF is further classified into following two types −

• Self-Induced EMF

• Mutually Induced EMF

Self Induced EMF

When EMF is induced in a conductor or coil due to change of its own magnetic flux linkage, it is known as self-induced EMF.

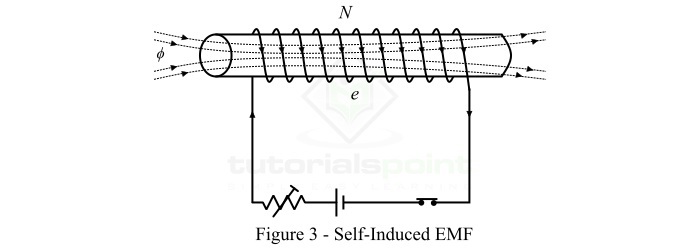
Consider a coil of N turn as shown in Figure-3. The current flowing through the coil establishes a magnetic field in the coil. If the current in the coil changes, then the magnetic flux linking the coil also changes. This changing magnetic field induces an EMF in the coil according to the Faraday’s law of electromagnetic induction. This EMF is known as self-induced EMF and the magnitude of the self-induced EMF is given by,

$$mathrm{mathit{e}:=:mathit{N}frac{mathit{dphi }}{mathit{dt}}}$$

Mutually Induced EMF

The EMF induced in a coil due to the changing magnetic field of a neighboring coil is known as mutually induced EMF.

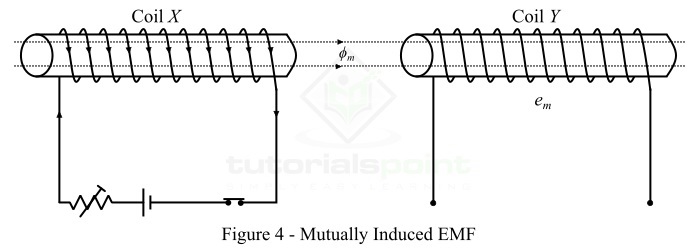
Consider two coils X and Y placed adjacent to each other as shown in Figure-4. Here, a fraction of the magnetic flux produced by the coil X links with the coil Y. This magnetic flux of coil X which is common to both coils X and Y is known as mutual flux ($mathit{phi _{m}}$).

If the current in coil X is changed, then the mutual flux also changes and hence EMF is induced in both the coils. Where, the EMF induced in coil X is known as self-induced EMF and the EMF induced in coil Y is called mutually induced EMF.

According to Faraday’s law, the magnitude of the mutually induced EMF is given by,

$$mathrm{mathit{e_{m}}:=:mathit{N_{Y}}frac{mathit{dphi _{m}}}{mathit{dt}}}$$

Where,$mathit{N_{Y}}$ is the number of turns in coil Y and $frac{mathit{dphi _{m}}}{mathit{dt}}$ is rate of change of mutual flux.