Simplification Using Algebraic Functions
In this approach, one Boolean expression is minimized into an equivalent expression by applying Boolean identities.
Problem 1
Minimize the following Boolean expression using Boolean identities −
$$F (A, B, C) = A”B + BC”+ BC + AB”C”$$
Solution
Given,$F (A, B, C) = A”B + BC”+ BC + AB”C”$
Or,$F (A, B, C) = A”B + (BC”+ BC”) + BC+ AB”C”$
[By idempotent law, BC’ = BC’ + BC’]
Or,$F (A, B, C) = A”B + (BC”+ BC) + (BC”+ AB”C”)$
Or,$F (A, B, C) = A”B + B(C”+ C) + C”(B+ AB”)$
[By distributive laws]
Or,$F (A, B, C) = A”B + B.1 + C”(B + A)$
[ (C” + C) = 1 and absorption law (B + AB”)= (B + A)]
Or,$F (A, B, C) = A”B + B + C”(B + A)$
[ B.1 = B ]
Or,$F (A, B, C) = B(A”+ 1) + C”(B + A)$
Or,$F (A, B, C) = B.1 + C”(B + A)$
[ (A” + 1) = 1 ]
Or,$F (A, B, C) = B + C”(B + A)$
[ As, B.1 = B ]
Or,$F (A, B, C) = B + BC” + AC”$
Or,$F (A, B, C) = B(1 + C”) + AC”$
Or,$F (A, B, C) = B.1 + AC”$
[As, (1 + C”) = 1]
Or,$F (A, B, C) = B + AC”$
[As, B.1 = B]
So,$F (A, B, C) = B + AC”$is the minimized form.
Problem 2
Minimize the following Boolean expression using Boolean identities −
$$F (A, B, C) = (A + B) (A + C)$$
Solution
Given, $F (A, B, C) = (A + B) (A + C)$
Or, $F (A, B, C) = A.A + A.C + B.A + B.C$ [Applying distributive Rule]
Or, $F (A, B, C) = A + A.C + B.A + B.C$ [Applying Idempotent Law]
Or, $F (A, B, C) = A(1 + C) + B.A + B.C$ [Applying distributive Law]
Or, $F (A, B, C) = A + B.A + B.C$ [Applying dominance Law]
Or, $F (A, B, C) = (A + 1).A + B.C$ [Applying distributive Law]
Or, $F (A, B, C) = 1.A + B.C$ [Applying dominance Law]
Or, $F (A, B, C) = A + B.C$ [Applying dominance Law]
So, $F (A, B, C) = A + BC$ is the minimized form.
Karnaugh Maps
The Karnaugh map (K–map), introduced by Maurice Karnaughin in 1953, is a grid-like representation of a truth table which is used to simplify boolean algebra expressions. A Karnaugh map has zero and one entries at different positions. It provides grouping together Boolean expressions with common factors and eliminates unwanted variables from the expression. In a K-map, crossing a vertical or horizontal cell boundary is always a change of only one variable.
Example 1
An arbitrary truth table is taken below −
| A | B | A operation B |
|---|---|---|
| 0 | 0 | w |
| 0 | 1 | x |
| 1 | 0 | y |
| 1 | 1 | z |
Now we will make a k-map for the above truth table −

Example 2
Now we will make a K-map for the expression − AB+ A’B’
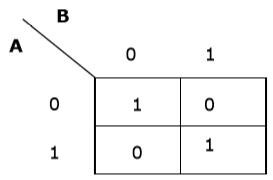
Simplification Using K-map
K-map uses some rules for the simplification of Boolean expressions by combining together adjacent cells into single term. The rules are described below −
Rule 1 − Any cell containing a zero cannot be grouped.
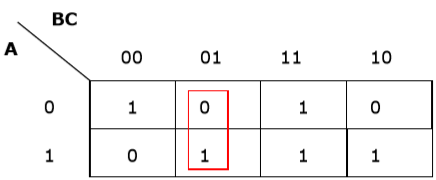
Wrong grouping
Rule 2 − Groups must contain 2n cells (n starting from 1).

Wrong grouping
Rule 3 − Grouping must be horizontal or vertical, but must not be diagonal.
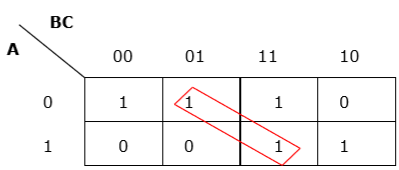
Wrong diagonal grouping
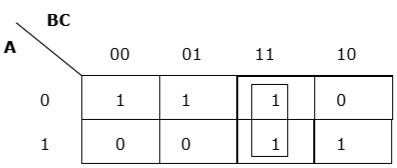
Proper vertical grouping
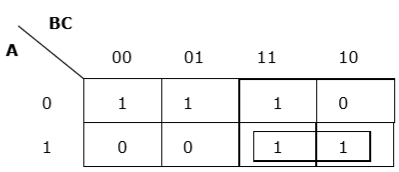
Proper horizontal grouping
Rule 4 − Groups must be covered as largely as possible.
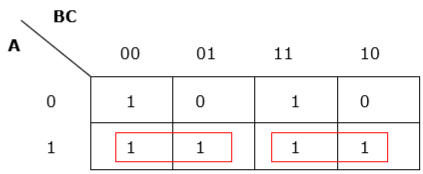
Insufficient grouping

Proper grouping
Rule 5 − If 1 of any cell cannot be grouped with any other cell, it will act as a group itself.
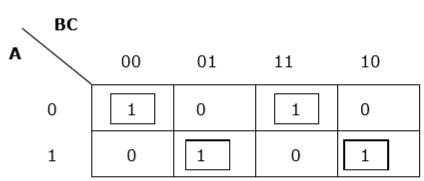
Proper grouping
Rule 6 − Groups may overlap but there should be as few groups as possible.
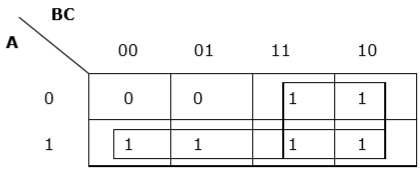
Proper grouping
Rule 7 − The leftmost cell/cells can be grouped with the rightmost cell/cells and the topmost cell/cells can be grouped with the bottommost cell/cells.
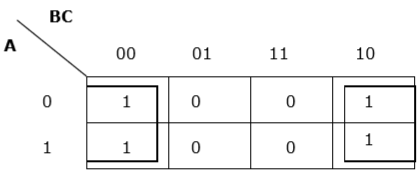
Proper grouping
Problem
Minimize the following Boolean expression using K-map −
$$F (A, B, C) = A”BC + A”BC” + AB”C”+ AB”C$$
Solution
Each term is put into k-map and we get the following −

K-map for F (A, B, C)
Now we will group the cells of 1 according to the rules stated above −
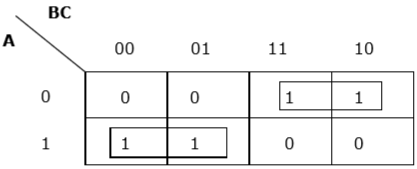
K-map for F (A, B, C)
We have got two groups which are termed as $A’B$ and $AB’$. Hence, $F (A, B, C) = A’B+ AB’= A oplus B$. It is the minimized form.
Learning working make money