In this chapter, we will derive the expression for mechanical power developed (P_m) by a three-phase synchronous motor. Here, we will neglect the armature resistance R_a of the synchronous motor. Then, the armature copper loss will be zero and hence the mechanical power developed by the motor is equal to the input power (P_in) to the motor, i.e.,

$$mathrm{mathit{P_{m}}:=:mathit{P_{in}}}$$

Now, consider an under-excited (i.e.,E_b<V) three-phase synchronous motor having zero armature resistance (i.e.,R_a = 0), and is driving a mechanical load.

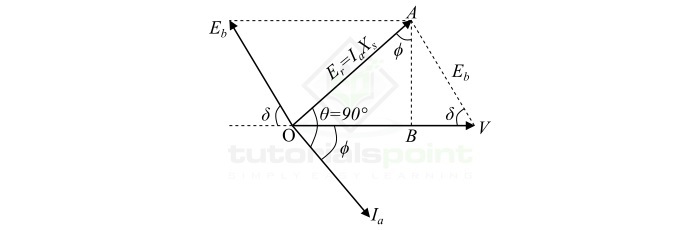
The phasor diagram of one phase of this synchronous motor is shown in the figure. Because the motor is under-excited, thus it will operate at a lagging power factor, say (cos $phi$). From the phasor diagram, it is clear that $mathit{E_{r}}:=:I_{a}X_{s}$ and the armature current per phase $I_{a}$ lags the resultant EMF $mathit{E_{r}}$ by an angle of 90°.

Therefore, the input power per phase to the motor is given by,

$$mathrm{mathit{P_{in}}:=mathit{VI_{a}}:cos:phi :cdot cdot cdot (1)}$$

Since $mathit{P_{m}}$ is equal to $mathit{P_{in}}$, therefore,

$$mathrm{mathit{P_{m}}:=mathit{VI_{a}}:cos:phi :cdot cdot cdot (2)}$$

From the phasor diagram, we have,

$$mathrm{mathit{AB}:=mathit{I_{a}X_{s}}:cos:phi :=:mathit{E}_{mathit{b}}:sin:delta}$$

$$mathrm{therefore mathit{I_{a}:cosphi :=:frac{E_{b}:sindelta }{mathit{X_{s}}}}:cdot cdot cdot (3)}$$

Using equations (2) & (3), we obtain,

$$mathit{P_{m}:=:frac{mathit{VE_{b}}sindelta }{X_{s}}}cdot cdot cdot (4)$$

This is the expression for mechanical power developed (P_m) per phase by the synchronous motor.

For 3-phases of the motor, the developed mechanical power is given by,

$$mathit{P_{m}:=:frac{3mathit{VE_{b}}sindelta }{X_{s}}}cdot cdot cdot (5)$$

Also, from the equations (4) & (5), it is clear that the mechanical power developed will be maximum when power angle ($delta$= 90°) electrical. Therefore,

For per phase,

$$mathit{P_{max}:=:frac{mathit{VE_{b}}}{X_{s}}}cdot cdot cdot (6)$$

For 3-phase,

$$mathit{P_{max}:=:frac{mathit{3VE_{b}}}{X_{s}}}cdot cdot cdot (7)$$

Important Points

The following important points may be noted about the mechanical power developed by a three-phase synchronous motor −

• The mechanical power developed by the synchronous motor increases with the increase in power angle ($delta$) and vice-versa.

• If power angle ($delta$) is zero, then the synchronous motor cannot develop mechanical power.

• When the field excitation of the synchronous motor is reduced to zero, i.e., ($E_{b}$ = 0), the mechanical power developed by the motor is also zero, i.e. the motor will come to a stop.

Numerical Example

A 3-phase, 4000 kW, 3.3 kV, 200 RPM, 50 Hz synchronous motor has per phase synchronous reactance of 1.5 $Omega$. At full-load, the power angle is 22° electrical. If the generated back EMF per phase is 1.7 kV, calculate the mechanical power developed. What will be the maximum mechanical power developed?

Solution

Given data,

• Voltage per phase, $mathit{V}:=:frac{3.3}{sqrt{3}}:=:1.9:kV$

• Back EMF per phase,$mathit{E_{b}}:=:1.7:kV$

• Synchronous reactance,$X_{s}:=:1.5Omega $

• Power angle,$delta :=:22^{^{circ}}$

Therefore, the mechanical power developed by the motor is,

$$mathrm{mathit{P_{m}}:=:frac{3:mathit{VE_{b}:sindelta} }{mathit{X_{s}}}:=:frac{3times 1.9times 1.7times mathrm{sin}:22^{circ}}{1.5}}$$

$$mathit{therefore P_{m}}:=:2.42times 10^{6}:W:=:2.42:MW$$

The mechanical power developed will be maximum when ($delta$ =90°),

$$mathit{P_{max}}:=:frac{3mathit{VE_{b}}}{X_{s}}:=:frac{3times 1.9times 1.7}{1.5}$$

$$mathit{therefore P_{max}}:=:6.46:mathrm{MW}$$